So natural minor scales are those that are in their non-transformed form?
In this tutorial, we are going to take a look at how minor scales are built. We will also discuss scale relativity. So, let's have some fun
2. Natural minor scales explained
Minor scales function in a similar manner to major scales. They also have 7 notes, which have to be located at certain tonal distances between one another in order to have a proper minor scale.
Let's take the A minor scale as an example. It consists of the A, B, C, D, E, F, G, and A notes. As a result, the formula for minor scales is the following one:
What that means is that the distance between the first note and the second note is one tone, the difference between the second note and third note is one semitone and so on.
The Am scale looks something like this:
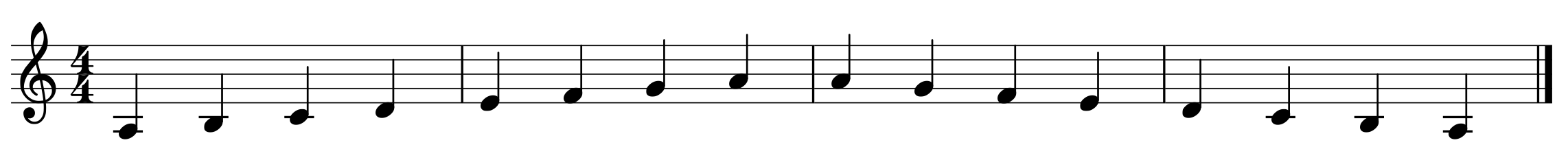
And here it is played back.
3. Scale relativity
Those of you with a keen eye will have noticed that the C major and A minor scale have the same notes. And this observation is correct.
When it comes to music, each major scale has a relative minor scale and vice-versa. And it's pretty easy to figure out the relative of a scale.
If you want to figure out the relative minor scale for a major scale, you have to look at the sixth note from the major scale. That note will be the starting note (or root note) for your relative minor scale.
If you want to figure out the relative major scale for a minor scale, you have to look at the third note from the minor scale. That note will be the root note of the relative major scale.
In our case, the sixth note of the C major scale is A. As a result, the A minor scale will be the relative minor scale for the C major scale.
Similarly, the third note of the A minor scale is C. As a result, the C major scale will be the relative major scale for the A minor scale.
That about covers it for this tutorial. In the next one, we are going to look at harmonic minor scales. See you then.
- Topics of discussion
- Natural minor scales explained
- Scale relativity
In this tutorial, we are going to take a look at how minor scales are built. We will also discuss scale relativity. So, let's have some fun
2. Natural minor scales explained
Minor scales function in a similar manner to major scales. They also have 7 notes, which have to be located at certain tonal distances between one another in order to have a proper minor scale.
Let's take the A minor scale as an example. It consists of the A, B, C, D, E, F, G, and A notes. As a result, the formula for minor scales is the following one:
Tone Semitone Tone Tone Semitone Tone Tone
What that means is that the distance between the first note and the second note is one tone, the difference between the second note and third note is one semitone and so on.
The Am scale looks something like this:
And here it is played back.
3. Scale relativity
Those of you with a keen eye will have noticed that the C major and A minor scale have the same notes. And this observation is correct.
When it comes to music, each major scale has a relative minor scale and vice-versa. And it's pretty easy to figure out the relative of a scale.
If you want to figure out the relative minor scale for a major scale, you have to look at the sixth note from the major scale. That note will be the starting note (or root note) for your relative minor scale.
If you want to figure out the relative major scale for a minor scale, you have to look at the third note from the minor scale. That note will be the root note of the relative major scale.
In our case, the sixth note of the C major scale is A. As a result, the A minor scale will be the relative minor scale for the C major scale.
Similarly, the third note of the A minor scale is C. As a result, the C major scale will be the relative major scale for the A minor scale.
That about covers it for this tutorial. In the next one, we are going to look at harmonic minor scales. See you then.